|
|
In class, we talked about a way to figure out the distance to the Moon using the triangle the moon makes with two points on Earth. Actually, it was just one point on Earth, at two different times an hour apart. One problem with this method is that the Moon moves a bit in its orbit over the course of this hour. The situation is like this:
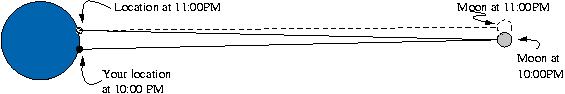
We want to find out how big a problem this movement is, and what we should do
about it. The diagram might help you with some of the the following questions:
1. We know that the Moon moves all the way around its orbit in 29.5 days.
a. How many hours is 29.5 days?
b. If it goes 360° in that many
hours, how many degrees does it go in just one hour?
Let's focus on the triangle made by the solid lines in the diagram, with vertices (corners) defined by the three points "Your location at 10:00," "Your location at 11:00," and "Moon at 10:00." This would be the scenario if the Moon weren't moving in its orbit. It turns out that the narrow angle in this triangle (the angle at the Moon) would measure about 0.25° if we could really go out and measure it by looking at the Moon in the sky. (it's drawn a little wider than that though, so don't bother measuring it on this paper!)
2. So if we pretend the Moon doesn't move, we'd be trying to measure an angle that's really about 0.25°. In part (a), you found out how big an error we'd have from ignoring the Moon's movement over an hour. Compared to 0.25°, is this error big enough to worry about?

3. Let's see if we can figure out what to do about this error by looking at this close-up of the diagram. One way to figure out the narrow angle in the diagram above is to subtract "Angle 1" from "Angle 2." That is, "Narrow angle" = "Angle 2" - "Angle 1."
(a) If we didn't know the Moon is moving in its orbit, we would want to measure Angle 2 according to the solid line, even though we'd really be measuring it according to the dotted line. So would the measurement we really get for "Angle 2" be bigger or smaller than the measurement we want?
(b) So would our calculation of "Narrow angle" end up bigger or smaller than it really should be?
(c) In question 1, you figured out how far off our calculation of "Narrow angle" would be, and in part (b) you just figured out whether our calculation is bigger or smaller than it should be. How would you fix the problem?